Physical Properties of Lanthanides – Inorganic Chemistry

Learn The Properties Of Lanthanides In Detailed Explanation

The 14 elements (Ce to Lu) are called the lanthanoids, or lanthanides. These elements are characterized by the filling up of the antepenultimate 4f energy levels. They are extremely similar to each other in properties, and until 1907 they were thought to be a single element. In the past, they were called rare earths. This name is not appropriate because many of the elements are not particularly rare. Furthermore, the name is not precise since in addition to the 14 lanthanides it has been used to include La, or Sc, Y and La which are d-block elements, and sometimes Th (an actinide) and Zr (another d-block element). Here we will learn the most prominent Properties of Lanthanides – Electronic Structure, Oxidation State, Solubility, Colour and Spectra, and last but not least the Magnetic Properties.

ELECTRONIC STRUCTURE

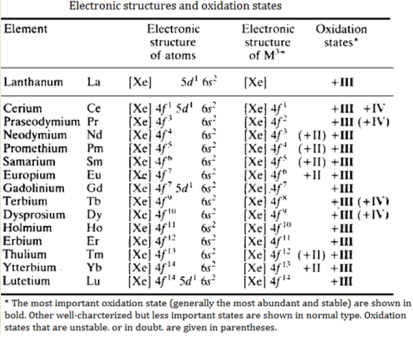
The electronic structures of the lanthanide metals are shown in Table. Lanthanum (the d-block element preceding this series) has the electronic structure: xenon core 5d¹ 6s². It might be expected that the 14 elements from cerium to lutetium would be formed by adding 1, 2, 3,.. .14 electrons into the 4f level. However, it is energetically favourable to move the single 5d electron into the 4f level in most of the elements, but not in the cases of Ce, Gd and Lu. The reason why Gd has a 5d¹ arrangement is that this leaves a half-filled 4f level, which gives increased stability.

Lu has a 5d¹ arrangement because the f shell is already full. The lanthanides are characterized by the uniform (+III) oxidation state shown by all the metals. They typically form compounds which are ionic and trivalent. The electronic structures of the ions are Ce³⁺ f¹, Pr³⁺ f², Nd³⁺ f³, . . Lu³⁺ f¹⁴.

The 4f electrons in the antepenultimate shell are very effectively shielded from their chemical environment outside the atom by the 5s and 5p electrons. Consequently, the 4f electrons do not take part in bonding. They are neither removed to produce ions nor do they take any significant part in crystal field stabilization of complexes. Crystal field stabilization is very important with the d-block elements. The octahedral splitting of f orbitals Δ_o is only about 1 kJ mol^-1. Whether the f orbitals are filled or empty has little effect on the normal chemical properties. However, it does affect their spectra and magnetic properties.

OXIDATION STATES – A General Property of Lanthanides

The oxidation state (+lll) is ionic and Ln³⁺ dominates the chemistry of these elements. The Ln²⁺ and Ln⁴⁺ ions that do occur are always less stable than Ln³⁺. (Here Ln is used to denote any of the lanthanides.) In just the same way as for other elements, the higher oxidation states occur in the fluorides and oxides, and the lower oxidation states occur in the other halides, particularly bromides and iodides. Oxidation numbers (+ll) and (+lV) do occur, particularly when they lead to:

1. a noble gas configuration. e.g. Ce⁴⁺ (f⁰)
2. a half-filled f shell. e.g. Eu²⁺ and Tb⁴⁺ ( f⁷)
3. a completely filled f level. e.g. Yb²⁺ (f¹⁴).

In addition (+II) and ( + IV) states exist for elements that are close to these states. Thus Sm²⁺ and Tm²⁺ occur with f⁶ and f¹³ arrangements and Pr⁴⁺ and Nd⁴⁺ have f¹ and f² arrangements. The (+lll) state is always the most common and the most stable. The only (+IV) and (+Il) states which have any aqueous chemistry are Ce⁴⁺, Sm²⁺, Eu²⁺ and Yb²⁺.

The lanthanide elements resemble each other much more closely than do a horizontal row of the transition elements. This is because the lanthanides effectively have only one stable oxidation state, (+III). Thus in this series, it is possible to compare the effects of small changes in size and nuclear charge on the chemistry of these elements.

SOLUBILITY

Salts of the lanthanides usually contain water of crystallization. Solubility depends on the small difference between the lattice energy and the solvation energy, and there is no obvious trend in the group. The solubility of many of the salts follows the pattern of Group 2 elements. Thus the chlorides and nitrates are soluble in water and the oxalates, carbonates and fluorides are almost insoluble. Unlike Group 2, however, the sulphates are soluble. Many of the lanthanides form double salts with the corresponding Group 1 or ammonium salts, e.g. Na₂SO₄Ln₂(SO₄)₃. 8H₂O and as these double salts crystallize well, they have been used to separate the lanthanides from one another.

COLOUR AND SPECTRA: The Magnificent Properties of Lanthanides

Many trivalent lanthanide ions are strikingly coloured both in the sold state and in aqueous solution. The colour seems to depend on the number of unpaired f electrons. Elements with (n) f electrons often have a similar colour to those with (14 – n) f electrons. However, the elements in other valency states do not all have colours similar to their isoelectronic 3+ counterparts.

Colour arises because of the light of a particular wavelength visible region. The wavelength absorbed corresponds to the energy required to promote an electron to a higher energy level. In the lanthanides, spin-orbit coupling is more important than crystal field splitting. In the spectra of transition metals, crystal fields splitting is of major importance. All but one of the lanthanide ions show absorptions in the visible or near-UV regions of the spectrum.

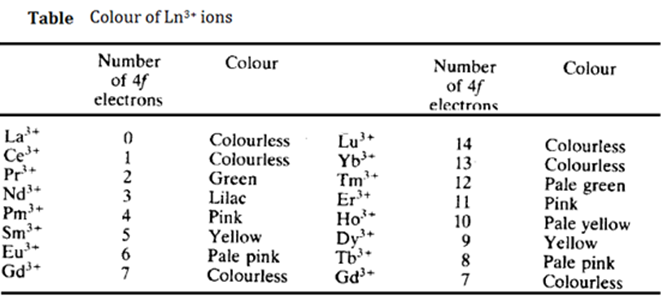
The exception is Lu³⁺ which has a full f shell. These colours arise from f-f transitions. Strictly these transitions are Laporte forbidden (since the change in the subsidiary quantum number is zero). Thus the colours are pale because they depend on the relaxation of the rule. The f orbitals are deep inside the atom. Thus they are largely shielded from environmental factors such as the nature and number of ligands which form the complexes, and from the vibration of the ligands. Thus the position of the absorption band (i.e. the colour) does not change with different ligands. The vibration of the ligands changes the external fields. However, this only splits the various spectroscopic states by about 100 cm^-1, so the absorption bands are unusually sharp. The lanthanides are used for wavelength calibration of instruments because of their sharp absorption bands.

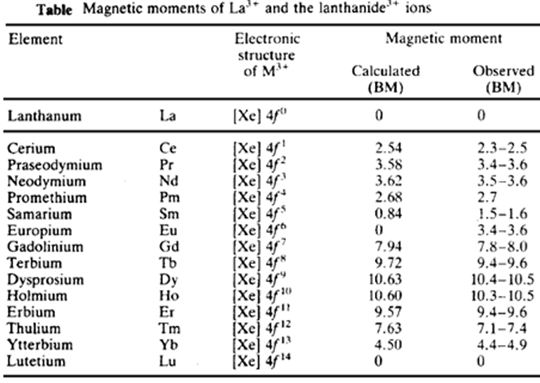
MAGNETIC PROPERTIES OF LANTHANIDES

La³⁺ and Ce⁴⁺ have an f⁰ configuration, and Lu³⁺ has an f¹⁴ configuration. These have no unpaired electrons and are diamagnetic. All other f states contain unpaired electrons and are therefore paramagnetic.

The magnetic moment of transition elements is calculated from the equation.

µ_{(S + L)} = √4S(S + 1) + L(L + 1)

(S + L) is the magnetic moment in Bohr magnetons. It is calculated using both the spin and orbital momentum contributions. S is the resultant spin quantum number and L is the resultant orbital momentum quantum number. For the first-row transition elements, the orbital contribution is usually quenched out by interaction with the electric fields of the ligands in its environment. Thus as a first approximation, the magnetic moment can be calculated using the simple spin only formula.

µ_S = √4S(S + 1)
µ_S = √n(n + 1)

This simple relationship works with La³⁺ (f⁰), and two of the lanthanides Gd³⁺ (f⁷) and Lu³⁺ (f¹⁴).

Magnetic Properties Of Other Lanthanides

The other lanthanide ions do not obey this simple relationship. The 4f electrons are well shielded from external fields by the overlying 5s and 5p electrons. Thus the magnetic effect of the motion of the electron in its orbital is not quenched out. Thus the magnetic moments must be calculated taking into account both the magnetic moment from the unpaired electron spins and that from the orbital motion. This also happens with the second and third-row transition elements.

However, the magnetic properties of the lanthanides are fundamentally different from those of the transition elements. In the lanthanides, the spin contribution S and orbital contribution L couple together to give a new quantum number J.

J = L – S When the shell is less than half full
J = L + S when the shell is more than half full

The magnetic moment µ is calculated in Bohr magnetons (BM) by:

µ = g√J(J + 1)

where

g = 1 + [S(S + 1) – L(L + 1) + J(J + 1)]/2 J(J + 1)